Find Out Your Cycle Times With Symbols
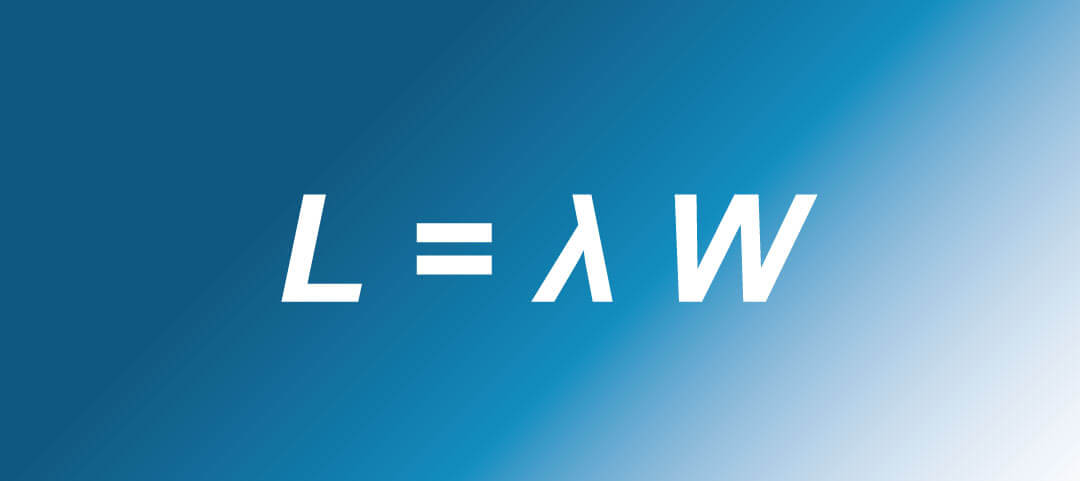
Time is Money
Cycle times affects the standard of your customer service, profitability, length of rentals and business reputation.
How can symbols help you find out your cycle time?
With the use of Little's Law.
So what is Little’s Law and what does it have to do with the collision repair business.
Let me start off by saying I had no idea what little’s law was so here is what I found out.
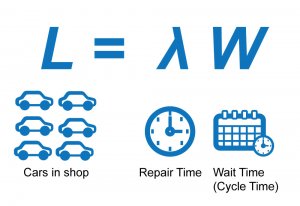
Essentially Little’s Law is a queueing theorem, that states the average number of items in a stationary system (L) is equal to the long term average arrival rate (λ) multiplied by the average time (W) that a item spends in the system. Algebraically the law is L = λ x W
Ok so that just hurt my head, so let's break it down a little and make some changes to the formula, we will see how it can relate to queuing systems in the collision repair industry.
L = Items in a stationary system to “Work in Progress” (WIP) - Total cars in the shop at a given time
λ = Long-term average arrival rate to “Throughput” - Average cars repaired per day
W = Time an item spends in a system to “Cycle time” - How long a customers car is in the shop
So Lets say currently you have 24 cars in your shop (Work in Progress (L)), your repair time is 4 cars per day (Throughput (λ)) so your cycle time (W) will be 6 days
The great thing about the theorem is that If you have any two values in the formula, you can easily calculate the third one:
Lead time = WIP / Throughput
Throughput = WIP / Lead time
Take a look at how this can be used in practice to improve workflow and productivity.
Let’s have a look at the example below:
You are a recognised Gold Class collision repair business with turnover of modern cars and the business is proving to be very popular due to their well trained workers and quick turnarounds.
Currently you have 24 cars in your shop at any given time (WIP (L)), your repair time is 4 cars per day (Throughput (λ)) so your lead time (W) will be 6 days
Let's say you improve your repair time due to well trained staff and now your repair time is at 6 cars per day this will reduce your lead time to 4 days ( 24/6 ) = 4
Alternatively let’s say your shop is getting an increase in cars waiting for repairs to 36 (WIP) at 4 repairs per day then your cycle time will increase to 9 days.
To reduce your cycle time back to 6 days you would have to increase your repair rate to 6 cars a day.
You can change these variables to other things like how many workers it takes to repair a car at a given amount of time and then look at increasing efficiencies.
Other areas you can apply the theorem to are:
Job scheduling for accessors / appraiser
Parts Handling
Paint and panel work
I-CAR Australia currently have two courses which relate to cycle times:
UNDERSTANDING CYCLE TIME AS AN APPRAISER AP055E02 :
Online training with post-test
UNDERSTANDING THE CYCLE TIME PROCESS GE001L01 :
Classroom & Virtual Classroom